# Simple Microscope (Elementary idea)

“It is a simple convex lens of short focal length used to see the magnified image of a small object.”

Principle:

When a small object is placed between optical centre and focus of a convex lens; a virtual, erect and magnified image of object is formed.

Ray diagram:

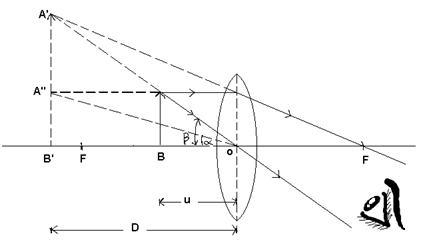
Magnifying  power (M) (Angular magnification)

M = Angle subtended by the image at the eye (or eye lens)/ Angle subtended by the image at the eye (or eye lens)

[When both are at least distance of distinct vision (D) from the eye]

[Imagine the object AB to be displaced to A”B’ at distance D from the eye]

( ∝, β are small)

In Δ ABO

tan β = AB/OB = AB/-u

In Δ A”B’O

tan ∝ = A”B’/OB’ = AB/-v

OB = -u ==>  object distance

OB’ = -v ==>  image distance    

∴ M ∝ 1/f

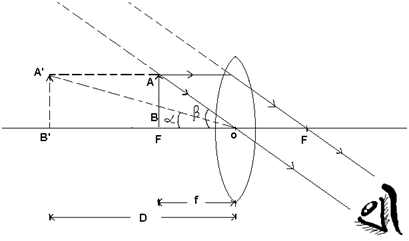
# Magnifying power when the image is at ∞

Magnifying power (M) = Angle made by the image (at ∞) at the eye/Angle made by the object at D

∴ M = β/∝ = tan β/tan ∝ = (AB/OB)/(A’B’/OB’) = (AB/OB)/(AB/OB’) = (OB’/OB) = (-D/-f) = D/f,         M = D/f

Uses of simple microscope:-

(i) Used by the watch makers and jewelers to have magnified images of small objects.

(ii) Used by the students in science labs for reading vernier scales etc.

# Compound microscope:-

It is an optical instrument used to see extremely small objects.

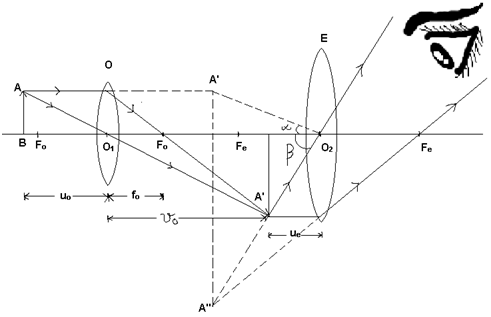
Construction:-

It consists of two convex lenses: An object lens O

 

 

Of small focal length and small aperture and an eye lens E of moderate focal length and large aperture. The two lenses are placed coaxially at the two ends of a tube. The distance between object lens and eye lens can be adjusted with the help of rack and pinion arrangement.

Principle:-

When a small object is placed between F and 2F of the object lens; its real, inverted and magnified image is formed. The image so produced acts as an object for the eye lens. The final image formed by the eye lens is virtual and very much magnified. The adjustments are so made that the final image is at the least distance of distinct vision (D) from the eye.

Magnifying power (M):-

[When both are at the least distance of distinct vision form the eye]

In Δ A”B”O₂:

tan β = A”B”/O₂ B”

In Δ A₁B”O₂:

tan ∝ = A₁B”/O₂B”

M = β/∝ = tan β/tan ∝ = (A”B”/O₂B”)/(A₁B”/O₂B”)

M = (A”B”/A₁B”) = (A”B”/AB)                        ( A₁B” = AB)

Or      M = (A”B”/A’B’) x (A’B’/AB)    (Multiplied & divided by A’B’)

Or      M = M_e x M₀                  —(1)

Where M_e = (A”B”/A’B’) → Magnification produced by eye lens

M₀ = (A’B’/AB) = Magnification produced by object lens

Now, M_e = (1 + D/f_e)

And M₀ = v₀/-u₀

∴ M = v₀/-u₀(1 + D/f_e)                       —(2)

Now, u₀ ≈ f₀              (∵ object AB is very close to F₀)

v₀ ≈ o₁ o₂ L → Length of microscope tube       (∵ Image A’B’ is very close to eye lens)

 

M = L/-f_o (1 + D/f_e)                —(4)

(ii) Distance between object & eye lens,       [L = v₀ + u_e]

# When the final image is formed at infinity:           [M = L/-f₀ x D/f_e]