# Lens Formula:

It is the between object distance (u), image distance (v) and focal length (f) of a lens. (1/f = 1/v – 1/u)

# Lens formula for convex lens:

(a) When the object lies between F and 2F (Real image is formed)

Let AB → Object place

A’B’ → Image formed

O → Optical centre of lens

 

 

F → Principal focus lens 

Triangle’s ABO & A’B’O are similar:

∴ (A’B’/AB) = OB’/OB = v/-u                           —(1)

Triangle’s ODE & A’B’F are similar:

∴ (A’B’/OD) = FB’/OF = OB’ – OF/OF = (v - f/f)

Or      (A’B’/AB) = (v – f/f)                               —(2)

From (1) and (2) (v – f/f) = (v/-u) or (v/f – 1) = (v/ -u)

Or       (1/f – 1/v = -1/u)     or        (1/f = 1/v – 1/u) (lens formula)

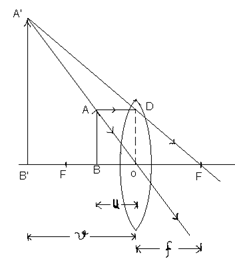
(b) When the object lies between O & F (Virtual image is formed)

Let AB → Object placed

A’B’ → Virtual image                                                       

Triangle’s ABO & A’B’O are similar:

∴ (A’B’/AB) = OB’/OB = -v/-u = v/u            —(1)

Triangle’s ODE & A’B’F are similar:

∴ (A’B’/OD) = B’F/OF = OB’ + OF/OF = -v + f/f

Or      (A’B’/AB) = -v + f/f                                —(2)

From (1) and (2) (-v + f/f) = v/u

Or       (-v/f + 1) = v/u

Or       (-1/f + 1/v = 1/u

Or       (1/f = 1/v – 1/u)

# Lens formula for concave lens:

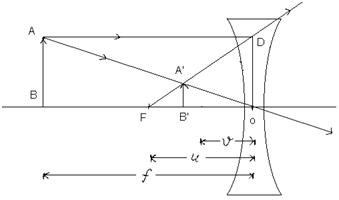
* When the object is placed between F & 2F:

Note: [Image formed by a concave lens is always virtual]

Let AB → Object placed

A’B’ → Virtual image

Triangle’s A’B’O & ABO are similar:                

∴ (A’B’/AB) = OB’/OB = -v/-u = v/u            —(1)

Triangle’s ODE & A’B’F are similar:

∴ (A’B’/OD) = B’F/OF = OF – OB’/OF = (-f – (-v)/-f)

Or      (A’B’/AB) = (-f + v/-f) = (1 – v/f)                 —(2)

From (1) and (2)

1 – v/f) = (v/u)          or        (1/v – 1/f = 1/u)      or        (1/f = 1/v – 1/u)