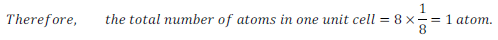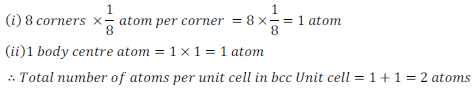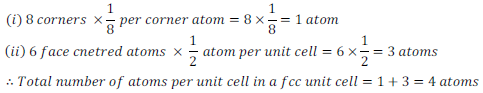Number of Atoms in a Unit Cell

A crystal lattice is made of very large number of unit cells and lattice points are the representation of constituent particles. Therefore, the number of atoms in a unit cell of a crystal lattice can be calculated.

Number of atoms in Primitive Cubic Unit Cell –

In primitive unit cell, atoms are present at corners only. In a crystal lattice every corner is shared by eight adjacent unit cells. Therefore, only 1/8 of an atom, or other constituent particles, belong to a particular unit cell.

Therefore,

Since, there are 8 atoms present in a unit cell on every corner,

Thus, 1 atom is present in a Primitive Cubic Unit Cell.

Body Centred Cubic (bcc) Unit Cell – There are eight atoms at each corner and one atom present at the centre of body in a body centred cubic (bcc) unit cell.

Therefore, the number of atoms present in a Body Centred Cubic (bcc) Unit Cell

Face – Centred Cubic (fcc) Unit Cell –

In a face centred cubic unit cell, there are eight atoms present at each corner. A cube has six faces, therefore total six atoms are present at the centre of each of the face.

Each atom present at corners is shared by adjacent eight atoms and each atom present at the centre of face is shared between adjacent two atoms.

Therefore, number of atoms in an fcc unit cell -

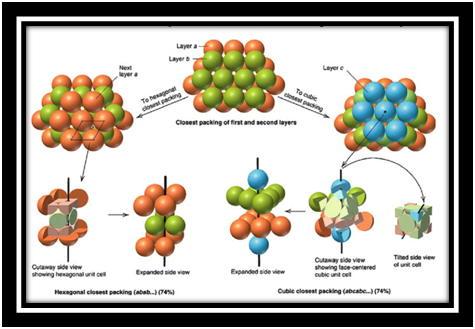
Close Packed Structure

Matters exist in solid state because of close packing of their constituent particles. There are two types of close packing found in solids. These are Cubic Close Packed (ccp) and Hexagonal Close Packed (hcp) lattice.

Cubic Close packed (ccp):

 In this type of packing, the spheres of molecules are adjacent to each other that each row of spheres in a particular dimension is a repetition of the pervious row. The spheres of a particular row don’t fit in the depressions between two adjacent spheres of the previous row. This types of arrangement is called AAAA type arrangement. This is also known as face centered cubic (fcc). This type of close packing of constituent particles is found in metals like copper, silver, etc.

Lattice of this cubic close packed is simple cubic and its unit cell is primitive cubic unit cell.

Hexagonal Close packed (hcp):

 In this type of packing, the spheres of molecules of a particular row in a particular dimension are in a position that they fit into depressions between adjacent spheres of the previous row. This type of arrangement is called ABAB type arrangement. This type of packed lattice is found in many metals such as magnesium, zinc, etc.

The following diagram depicts the formation of packing structures of different types of packing structures from different layers with their respective cut away side view and expanded side views respectively.