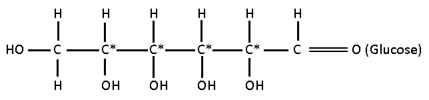
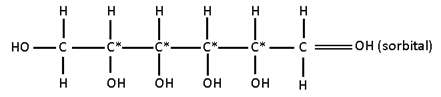
Possible StereoIsomers
NUMBER OF POSSIBLE STEREOISOMERS IN COMPOUNDS CONTAINING DIFFERENT NUMBER OF ASYMMETRIC ATOMS
The number of optical isomers is calculated by the application of the following rules:
The molecule has no symmetry (unsymmetrical) and (n) is the number of asymmetric carbon atoms, then
The number of d- and l- (optically active) forms, a = 2n
and The number of meso – forms, m = 0
Total number of optical (stereo) isomers = a + m = 2n
For example, CH3—CHOH—COOH (Lactic acid)
Where, n = 1, a 21 = 2, m = 0, r = (2/2) = 1
So, total optical isomers = 2 + 0 = 2.
(ii) When the molecule can be divided into equal halves, i.e., the molecule has symmetry and the number (n) of asymmetric carbon atoms is even, then
The number of d- and l- (optically active) forms, a = 2(n-1)
and The number of meso – forms, m = 2(n/2)-1
Total number of optical isomers = a + m
= 2(n-1) + 2(n/2)-1
For example, HOOC – *CHOH – *CHOH – COOH
Where, n=2, a=2n-1=22-1=21=2
m=2(n/2) – 1 = 2(2/2) – 1 = 21 – 1 = 1
So, total optical isomers = 2 + 1 = 3.
(iii) When the molecule can be divided into two equal halves (Symmetrical) and the number (n) of asymmetric carbon atoms is odd, then
The number of d-and l-forms, a = 2(n-1) – 2(n-1)/2
And The number of meso-forms, m=2(n-1)/2
Total number of optical isomers = a + m = 2(n-1)
For example,
CH2OH – *CHOH – *CHOH – *CHOH – CH2OH
Where, n=3, a=2(n-1) – 2(n-1)/2 = 22 – 21 = 2
m = 2(n-1)/2 = 2(3-1)/2 = 21 = 2
So, total optical isomers = 2 + 2 = 4
In all the above three cases, the number of racemic forms will be = a/2.
I) HOCH2 – (CHOH)4 – CHO
II) HOCH2 – (CHOH)4 – CH2OH
 SureDen
SureDen