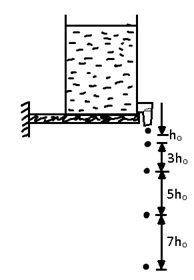
1. If water drops are allowed to fall from a certain height at regular intervals, then the distance between two successive drops are always in the ratio 1: 3 : 5 : 7 : 9 …………….

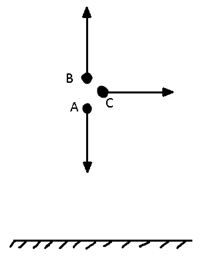
2. If three balls are thrown with same speed such that

• One is thrown vertically download (ball A)
• The second is thrown vertically upward (ball B), and
• The third is thrown horizontally (ball C),

Then all the three balls reach the ground with same speed.

If t_A, t_B and t_C are the respective time taken by the balls A, B and C, then 

NOTE If we consider a constant retarding force due to air resistance, then the ball taken less time to reach the highest position and larger time or reach the ground as compared to that in the absence of air resistance.

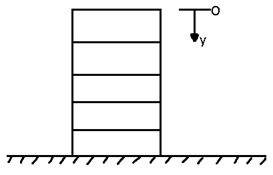
3.  A balloon stairs rising upward with constant acceleration a and after t_o second a packet is dropped from it which reaches the ground after t second. Determine the value of t.

Solution:

Assuming origin at the ground we have

y_o = + ½ at²₀; v_o = +at_o; g = -g; y = 0

Substituting the above values in the equation

y = y_o + v_ot + ½ gt²

we get

0 = ½ at_o² + at_ot – ½ gt₂

Or t² – (2at_o/g) - (a/g)t_o² = 0

Solving the quadratic equation, we get

4. A ball is dropped from the top of a building at t = 0. At a later time t = t_o, a second ball is thrown downward with initial speed v_o. obtain an expression for the time at which the two balls meet.

Solution:

Let us assume the origin at the top of the building with downward direction positive.

If the two balls meet at time t, then

y₁ = y₂

½ gt² = v_o(t – t_o)+ ½ g (t – t_o)²

After simplifying and solving, we get