When a body moves through a fluid, the fluid in contact with the body is dragged with it. This establishes relative motion in fluid layers near the body, due to which viscous force starts operating. The fluid exerts viscous force on the body to oppose its motion. The magnitude of the viscous force depends on the shape and size of the body, its speed and the viscosity of the fluid. Stokes established that if a sphere of radius r moves with velocity v through a fluid of viscosity h, the viscous force opposing the motion of the sphere is

F = 6phrv

This law is called Stokes law.

If a spherical body of radius r is dropped in a viscous fluid, it is first accelerated and then it's acceleration becomes zero and it attains a constant velocity called terminal velocity.

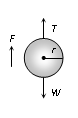
Force on the body

(i) Weight of the body (W) = mg = (volume × density) × g

(ii) Upward thrust (T) = weight of the fluid displaced

                                           = (volume × density) of the fluid × g

(iii) Viscous force (F) =

When the body attains terminal velocity the net force acting on the body is zero. W–T–F =0  or  F= W – T

(i) Terminal velocity depend on the radius of the sphere so if radius is made n - fold, terminal velocity will become n² times.         

(ii) Greater the density of solid greater will be the terminal velocity

(iii) Greater the density and viscosity of the fluid lesser will be the terminal velocity.

(iv) If r > s then terminal velocity will be positive and hence the spherical body will attain constant velocity in downward direction.

(v) If r < s  then terminal velocity will be negative and hence the spherical body will attain constant velocity in upward direction. Example : Air bubble in a liquid and clouds in sky.

(vi) Terminal velocity graph :